In today’s post I just might have figured out the fundamental angle of reflection, or the angle of bending of the two rings where each neighboring dot has the same length of separation at the instance of reflection. You remember, relating to this picture:
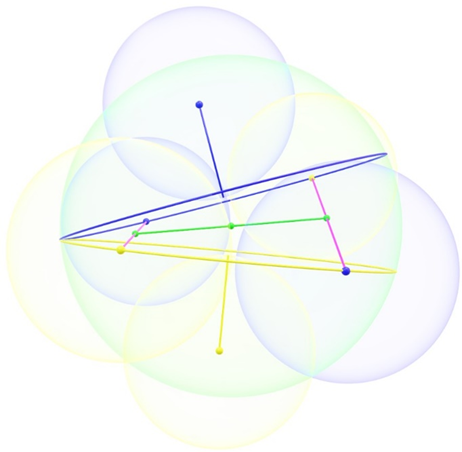
I’ve been writing equations from the above shape for a day since my last post, and I think I have the equations that define the distances between two neighboring dots in the above shape. I won’t show you the individual equations yet, but if one assumes that both equations are identical, you can subtract one equation from the other and divide by the radius of a dot and you get this equation:
With large values of θ the left side is large and with θ approaching zero the left side approaches zero from the wrong side. For an instant I was confused about this, because this seemed really odd. When approaching zero, the left term suddenly acquires a negative sign.
And how did I found this? By making series with the above equations with Excel, where the right term was x, instead of zero. I started by θ = 10 degrees, or 0.17 radians, and started dividing the angle by ten at each step. However, between θ = 10^-14 and 10^-15 the sign of the left term turned negative. Then I started dividing not by 10, but by 1.1, until I hit a similar sign reversal. Then I started dividing by 1.01, 1.001, 1.00001, 1.0000001 and even 1.00000000001, until I reached an angle of 6.4130054434 ∙10^-17 radians and thought that this is good enough accuracy for now. Going from this number to 6.4130054433 ∙10^-17 something odd happens. The sign reverses and the size of the term instantly reduces by seventeen orders of magnitude, from 1.77 ∙10^-15 to -1.65 ∙10^-32. If we consider the radius of the dot to be Planck length, and the full orbital of dots to be 2π radians, then the number of dots in the orbital would be 2π divided by the angle. When this number is multiplied by Planck length, we get a radius of roughly 1.6 10^-18 meters, which happens to be of the magnitude of the upper bounds of the radius of an electron. Whether this is just an accident, or something very fundamental, remains to be seen.
But as I tend to say, these are too early days to make grand proclamations. There are two things that I worry about. Firstly, as always, I fear that I’ve made an error in my calculations and the results above are just an error on my part. Secondly, Excel can be very unpredictable at very small and very large numbers. If at any point in the calculations, Excel reaches 2.225 × 10⁻³⁰⁸ or lower (at least according to ChatGPT), this can cause calculation errors. So don’t be surprised to find me posting that you can ignore this post altogether.
But at least for now, I’ve found a way to balance my shape and a very small angle that might, or might not, mean something extremely important to the Theory of Everything. But the only way for me to know this is to continue with this quest and for you, dear reader, to follow my posts. And whether or not the above angle is the fundamental angle of reflection, remains to be seen…
Comments