My Twisted Butterfly
- Kalle Lintinen
- Oct 8, 2023
- 3 min read
In my last post I warned that I might need to take a pause in my posts, as next I might just need to write the corrections to my Theory of Everything manuscript. However, I also said that if I figured out something new, I would sure keep you informed.
Well, as it happens, I really did figure out something new and really fast at that. You see, my manuscript had relied on the concept of there being two levels of twist in matter. The first level of twist entangles two helices of elementary particles into one entangled cord. But the second level of twist entangles this cord around itself. This was the basic idea of the manuscript until just about now.
However, almost as soon as I had the equations for the twisting of the entangled cord into a hydrogen orbital, I realized there was something off. The equations showing the second level of twist seemed to hold for charged particles, such as electrons and protons, but it didn’t make sense for the hydrogen atom.
To cut a long story short, applying the parametric equation resulting in double helices for the four petals of hydrogen, I was left with a term 2/4 in the equations. While this term might not ring alarm bells to anyone who hadn’t worked with the equations for months, I realized that the denominator in the term being even means that the equations no longer give a double helix. And without a double helix, there is no twisting. Or there isn’t any second-level twisting, to be more precise.
What this means is that while my equations were perfectly fine for charged particles, they were at least a bit off for non-charged particles. The good thing about having almost correct equations is that they can be used to find the fully correct ones. So, this is what I’m doing at the moment.
Just like with my twisted double helices, I began by making an Archimedean curve with a specific number of turns in Blender. This time that number was four. Then, as always, I bent the curve around itself by 720 degrees. After that I made an Archimedean curve with many more turns (100 to be specific), with a much smaller radius for each turn. The radius to height ratio of the turns I knew from earlier curves. Once the curve was ready, I made it follow the four-petal curve to get the first helix of the entangled state. Then I repeated the process but twisted the curve by 180 degrees around its axis to get the second helix of the entangled state. By giving the twists two color, I can illustrate the shape, like this:
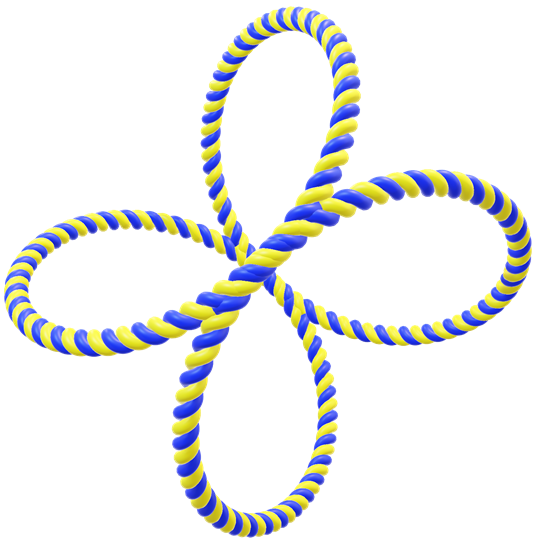
The more keen-eyed readers might notice that the loops in the curve overlap. This is what I meant when I said that my equations were almost correct. If I want them to be fully correct, I will need to find a way to account for the volume of the dots that make the curve up. Of course, I can always try to get the manuscript through without the exact mathematics. This is also an option, if the mathematics prove to be too hard for me.
However, I already know that I need to make the cone of refraction version of theabove twisted butterfly, as I did for the doubly twisted circle, as seen below.

I think if nothing else this combination of butterflies on two scales should make a very pretty figure.
And here is a side view of the twisted butterfly, just for the fun of it. From this image you might observe that the shape is a bit lop-sided. Apparently, it’s because with a small aspect ratio of the radius of this loop to the radius of the dots making this loop, the shape is not really symmetrical. Only with a high aspect ratio, the shape becomes symmetrical and pretty. However, a large aspect ratio is bad for illustration, because you no longer properly see the individual turns in the image. So it’s better to exaggerate for highest visual impact.

And here is an update from the same day:
I haven’t yet managed to add the cones of refraction, but switching the thick cord with an array of dots was easy-peasy. I still haven’t figured out how to resolve the problem of the curves overlapping, but I’m sure I’ll figure that out as well.

And here the same image viewed from the side:






Comments