Flyby Cones
- Kalle Lintinen
- Dec 29, 2024
- 2 min read
In my last post, ”Chiral Caterpillars”, I presented the first glimpse of the way four chiral versions of the unit sphere of reflection are connected to present a uniform model of reflection of elementary particles of energy (kaus/dots). The idea seemed good, but I already told in the post that I would need to check the theory for holes before making any pronouncements about it being the final solution to the theory of everything. Conversely, I said:
It almost seems more probable that there are at least a few holes left. I’ve almost never gotten an idea that was fully correct from the get-go.
And in today’s post I’m revealing at least some of these holes and introduce corrections to account for them.
The most critical of these holes are the points of reflection in the model and how they influence the shape of the unit spheres of reflection. I fear I won’t be able to explain this in an easy manner, but I’ll give it a try. Looking at the four chiral unit spheres of reflection, I noticed that there are only two nodes of reflection in the connection between at least two of the unit spheres. This seems to indicate that when you mirror two otherwise identical unit spheres of reflection, only the flyby paths on the opposite side of the hinge can end in reflections, whereas the flyby paths on the hinge side should pass the mirroring point with the direction unchanged. This means that the flyby angle should be zero on the hinge side. This might sound like an impossible task at first but is actually very easily solvable by rotating the hinges backwards by the inner flyby angle φ from the last post, reducing the hinge flyby angle to zero and the opposite flyby angle to γ – φ, where γ is the outer flyby angle without rotation. This rotation is seen as the projection of the hinge torus below projection to thicken from a line to a thin ellipse.
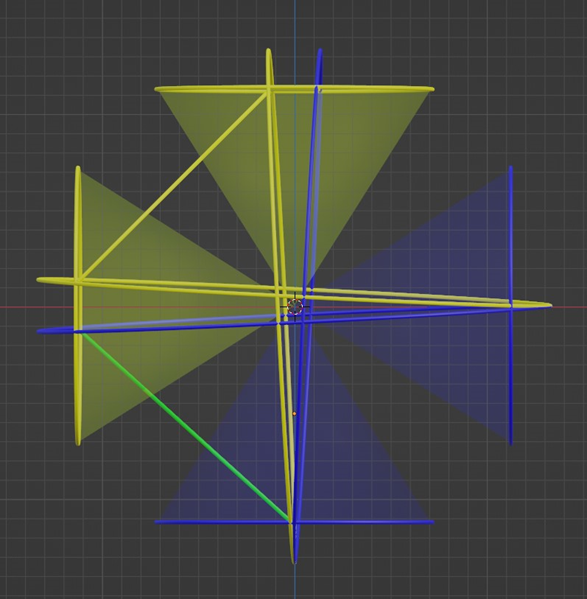
And you can see the same thickening of the torus around the yellow flyby path. This comes from the fact that the yellow flyby paths need to be tilted towards the mirroring point, the point where the kaus go through the proverbial looking glass. As the flyby paths are present at a specific distance from the center of the unit sphere, the torus around the flyby path can be connected to the center with a cone. This cone isn’t 100 % necessary for the illustration, but it helps to show how the flyby paths are rotated around two axes.
Here is a preliminary 3D model of the idea:
And as always, I remind you that the idea might still be proven incorrect. I still have at least one rather major unanswered question remaining that I don’t have the time to address in this post. It can either be very easy to solve, or possibly an incredibly hard task. I can prove or disprove the former hypothesis soon enough.





Comments