Yes, Strings Attached
- Kalle Lintinen
- Oct 25, 2023
- 3 min read
Updated: Oct 26, 2023
Today I’m going to share with you my first 3D-musings of the way monolignol toroidosomes entangle into a hexagonal lattice.
The curious thing is that I just realized that I had the answer to the rather complex structure of the lignin lattice in double helices. But back years ago, when I first had the Eureka moment about the structure of lignin, I didn’t have a clue it involved double helices.
However, rather than try to explain what I thought, to come up with this realization, I’ll just show you the images. Or rather I’ll show the end result. First of all I had a vague idea that the monolignol donut, or ‘toroidosomes’ would need to be fused together to form a uniform lattice. I already have a decent idea what the lattice would be like, if the monolignols would be present as independent rings. Because with rings you can consider each loop to be a separate entity, your really don’t need to worry about topology or similar difficult mathematical concepts.
Anyhow, almost immediately that I started pushing the donut-helix of monolignols together, I realized they need to form a double helix. It was obvious. After that the only thing that was left was to twist and turn helices until I got a structure that looked decent. Like this:
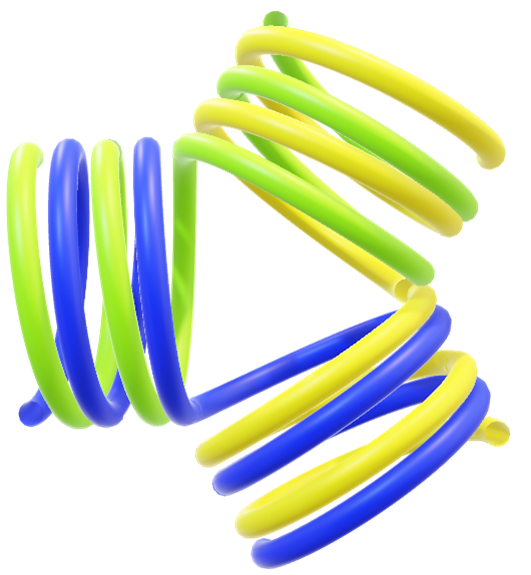
But this looked a bit wonky, because the initial state from which I started was a bit chaotic. So I tidied the structure up to look like this:

Which is almost identical, but more compact. After that I realized you can illustrate the structure of the entangled monolignol toroidosomes as six interconnected double-helical cylinders like this:
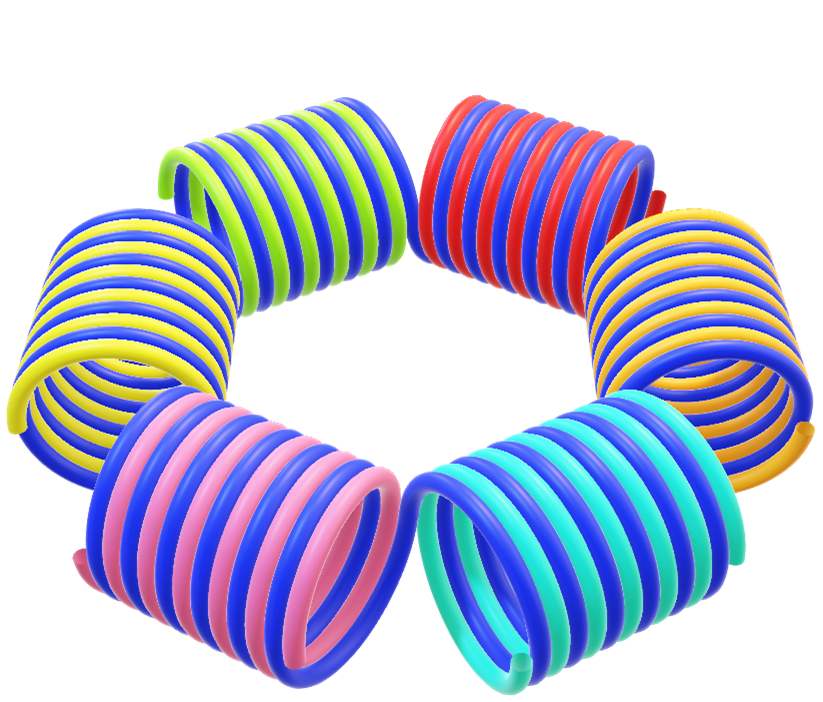
And if you remove the second helix of the double helix, you get a hexagonal helix like this:
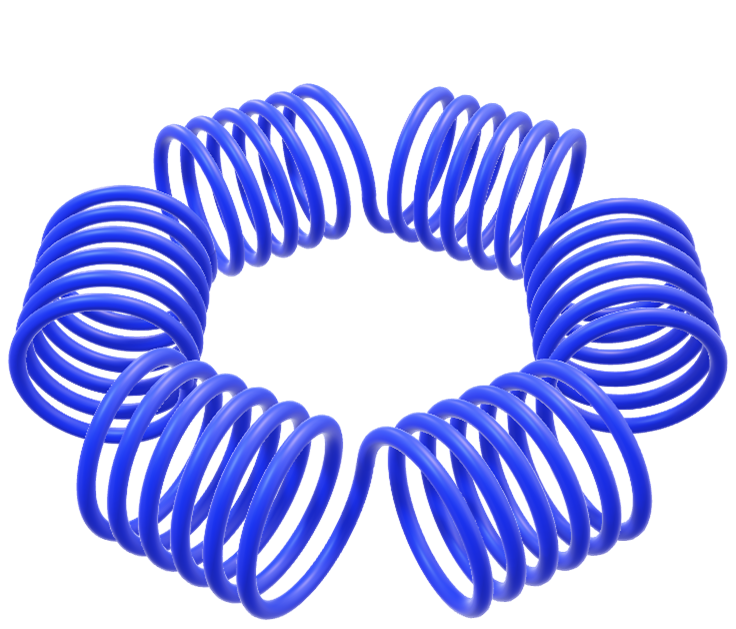
And you can tile this hexagonal helice into a hexagonal lattice like this:
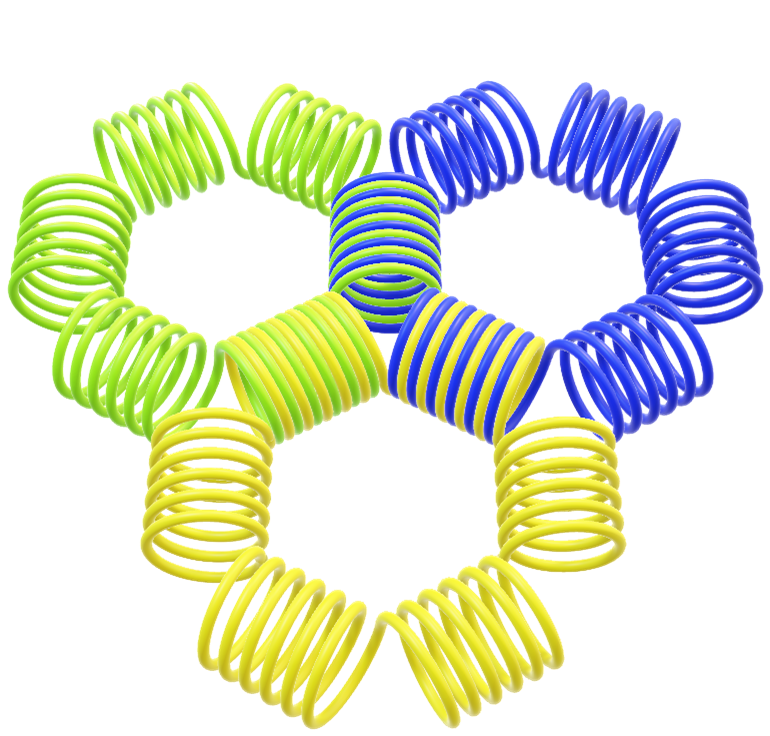
And if you consider that the double-helical tubules aren’t really hexagonal, you can deform each turn of the helix to get a shape pretty much like I envisioned:
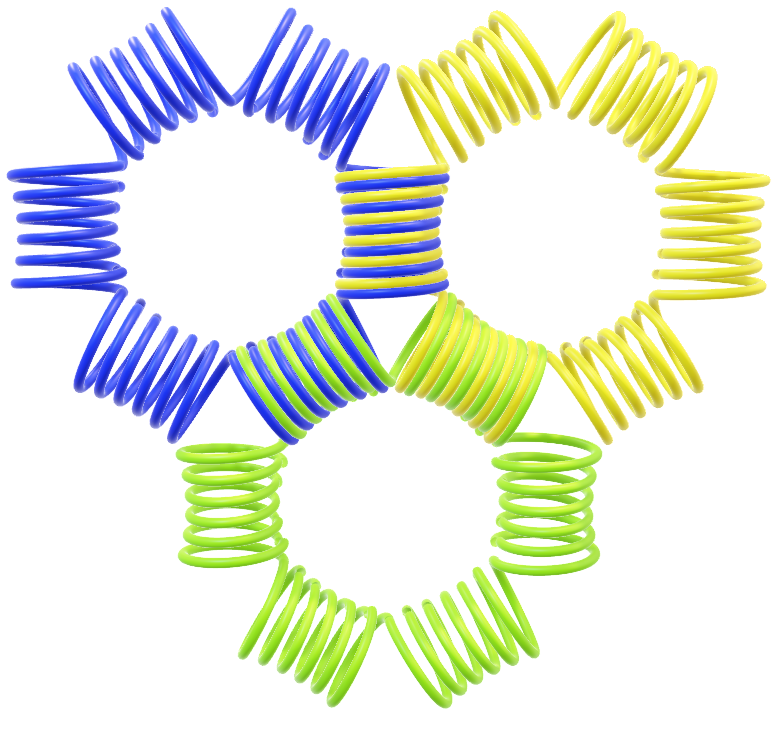
And here is the same shape at an angle:
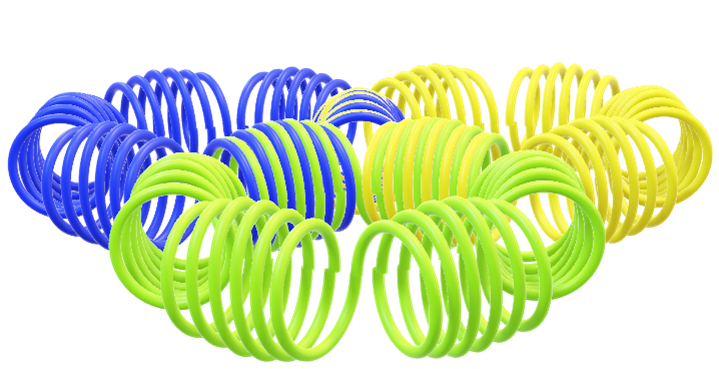
You can see that the deformation isn’t perfect yet. But the concept is sound.
Again, what you probably see are just geometrical shapes, but for me they are the structure of plant life. With double helices, just like with all life.
And these nanotubules forming hexagonal lattices isn’t just a random guess. I can see them with an electron microscope (albeit after quite a bit of processing).

This, my friends, is the Theory of Everything in action! I can’t say no strings attached, so I say “Yes, strings attached”.
Update from the next day:
After a night of sleep I decided to get back to the final deformed lattice, because the way I drew the structure in Blender led to a slight misalignment of the helices.
I already knew what the problem was: I had put two different sized helices together, whereas in real life there would be just one helix, with its radius gradually growing/reducing.
The easiest thing to introduce growing/reducing is to make an archimedean curve with a non-zero radius growth. This is the same shape I've used before. With such a shape, I was able to make a deformed hexagonal lattice in no time at all:
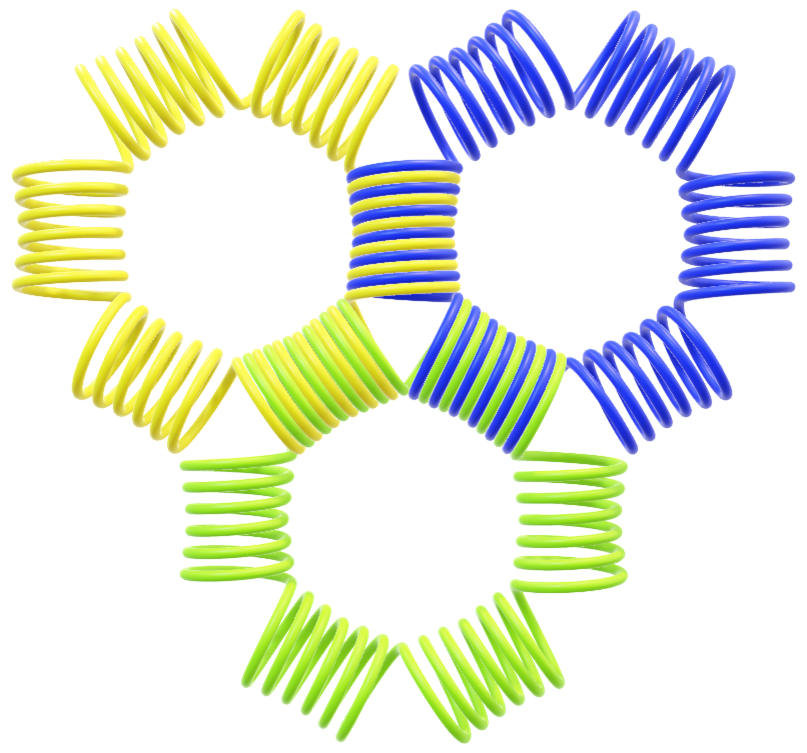
And here is the same shape at an angle:
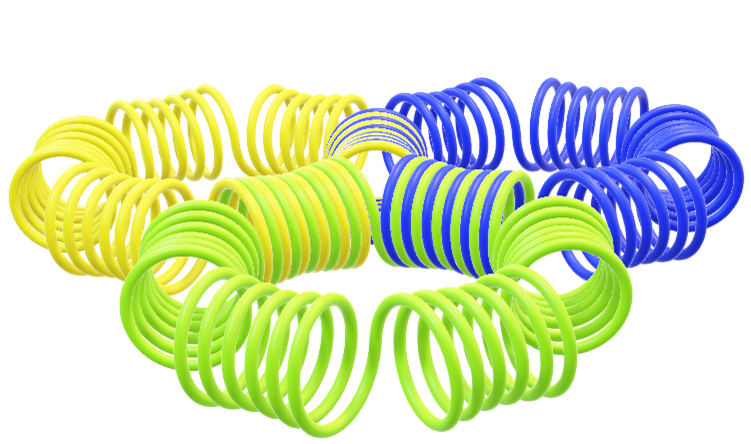
A bit like with the structure of the hydrogen orbital, the initial structure that I showed in yesterday's post might be closer to reality, but that would require a much more complex equation. For a publication, being able to show a uniform closed curve is even more important than being as close to reality as possible, if this 'real-world-model' assumes that a miracle occurs.
This means that just like with the Theory of Everything -manuscript, I might have to omit the 'truer' hypotheses in favor of the more approximate ones, where I can write equations for the shapes. There will come time for the truer shapes once the basic shapes have been accepted.
But I actually do have a hypothesis for the more complex shape: the helix comprises of quarter-turn-segments, with alternating positive and negative growth rates. The problem with this hypothesis is that I can't easily verify it with Blender. Blender has this annoying limitation that there have to be a whole number of turns. I can weasel myself out of this, but cutting three quarters of a single turn 'manually', but this is quite tedious. Let's see where I end up.





Comments